
This week we are happy to have Kyle Koppenhoefer of AltaSim Technologies as a guest blogger. As COMSOL Certified Consultants, AltaSim can share valuable insights into using the software — today on the topic of meshing wave problems.
As part of our efforts to assist COMSOL users in performing high-quality analysis, we recently used our bi-monthly email to remind users to include twelve degrees of freedom per wavelength when meshing wave problems. This article builds on our previous advice. Wave-type problems develop in a variety of analyses that include acoustics, electromagnetics, and structural vibrations. Thus, this mesh refinement issue touches many different industries.
Wave Problems Need Twelve Degrees of Freedom
Wave propagation problems require twelve degrees of freedom per wavelength to accurately represent the wave. This requirement develops from the error associated with approximating a sine wave with a polynomial. Twelve degrees of freedom provide a solution with less than 1% deviation from the approximated sine wave. These degrees of freedom may be obtained by using linear, quadratic, or cubic elements with fewer elements needed for the higher order elements.
Mesh Refinement How-to
To determine a proper mesh size, the analyst must know the wavelength of the problem that they are attempting to solve. The wavelength is the ratio of the wave speed to the frequency. For radio frequency problems, wave speed is inversely proportional to the refractive index of the media. For the case of mechanical wave propagation in solids and gases, wavelength depends on the density and bulk modulus of the material.
In problems with inhomogeneous media, the analyst should account for changes in wavelength within the solution domain. For example, speed of sound in the atmosphere depends on elevation. Thus, the mesh density should change with elevation.
A mesh refinement study provides valuable information and confidence in solution accuracy. Thus, solve the problem using multiple mesh densities to determine how the solution changes with increasing degrees of freedom. A demonstration of how to set up a simple wave propagation problem and the effects of the number of degrees of freedom on the solution can be found on the AltaSim Technologies Blog.
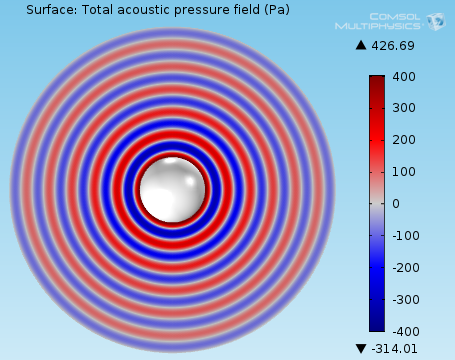
Wave propagating from pulsing sphere.
About the Guest Author
Kyle C. Koppenhoefer, Ph.D., has more than twenty years of experience working with computational analysis. He and Jeffrey Crompton started AltaSim Technologies, LLC eleven years ago to assist in the design of new products through the application of advanced computational tools. To facilitate this goal, AltaSim Technologies became a founding member of the COMSOL Certified Consulting program, and continues to be a leader in providing COMSOL services to people interested in receiving the benefits of modeling with COMSOL Multiphysics. AltaSim Technologies maintains a blog on their website, which provides their thoughts on multiphysics modeling.



Comments (0)