
Pole vaulting is one of the most difficult events to master in track and field. Athletes must be able to run fast, be strong enough to elevate their body by holding the pole, and have excellent body control in order to change position while airborne. Analyzing the science behind this sport offers greater insight into the mechanisms that ensure success.
The Different Phases of Pole Vaulting
Pole vaulting is a sport with a storied history. What began as an ancient competition for Greeks, Celts, and Cretans has evolved into a medaled event in the Olympic Games. Several tournaments, including the upcoming IAAF World Indoor Championships, are also hosted throughout the year, giving pole vaulters the opportunity to showcase their skills.
The sport itself, recognized as one of the major jumping events, involves the use of a long, elastic pole to clear a bar. In the past few decades, carbon fiber and fiberglass poles have arrived on the pole vaulting scene. These advancements are helping to bring athletes to new heights and break previous world records. While the pole has an important impact on performance, there are many other elements to consider that can affect the overall jump.
When it comes to clearing a height in pole vaulting, the general approach taken by athletes can be broken down into a series of phases. Each of the phases, listed here, places different constraints on the body:
- Run up
- Pole plant and takeoff
- Pole bend and swing
- Pull and release
- Clearance
In each phase, athletes control several of the initial conditions. Such conditions include: speed; grip height (the height at which the pole vaulter grips the pole); stiffness, which differs between different pole categories; the angle of attack (the angle between the pole and the ground at takeoff); and body position while airborne.
Angelica Bengtsson sets the Swedish pole vaulting record in 2015, achieving a 4.68 m clearance. Later that year, Bengtsson increased the national record to 4.70 m and finished in 4th place in the 15th IAAF World Championships.
Here, we’ll provide some more details about the individual phases.
Run Up
The run up phase refers to when an athlete holds the pole in an upright position and successively tilts it forward while approaching the box, the hole in the runway where the pole is placed. By holding the pole close to the body, the torque created by the weight of the pole decreases. The muscular strength thus becomes less fatigued, with most of the muscular energy retained in the body. While approaching the box, the athlete maximizes his or her speed in order to maximize the kinetic energy, EK, which is transferred to the next phase.
Pole Plant and Takeoff
During pole plant and takeoff, the pole is initially placed in the box. The athlete then bends the pole and jumps up. What we have here is a multibody system, a combination of the pole itself and the pole vaulter. To get the pole into a vertical position, the system must rotate forward. Several variables can affect the angular position of the pole, θ, including the jump force, F; the jump velocity, v; and the body mass, m.
The jump force is transferred through the body to the pole at the hand grip. This pole force creates a forward-rotating torque at the takeoff and provides a positive contribution to the forward rotation. The athlete’s velocity affects the angular momentum, which further adds to the forward rotation. The body mass, assisted by the gravity, g, creates a counteracting gravitational torque throughout the entire movement that decelerates the rotation. Additionally, the pole vaulter rotates around the hand grip, φ, and moves his or her body parts. Such motion alters the position of the body mass and the rotational inertia, influencing the pole rotation.
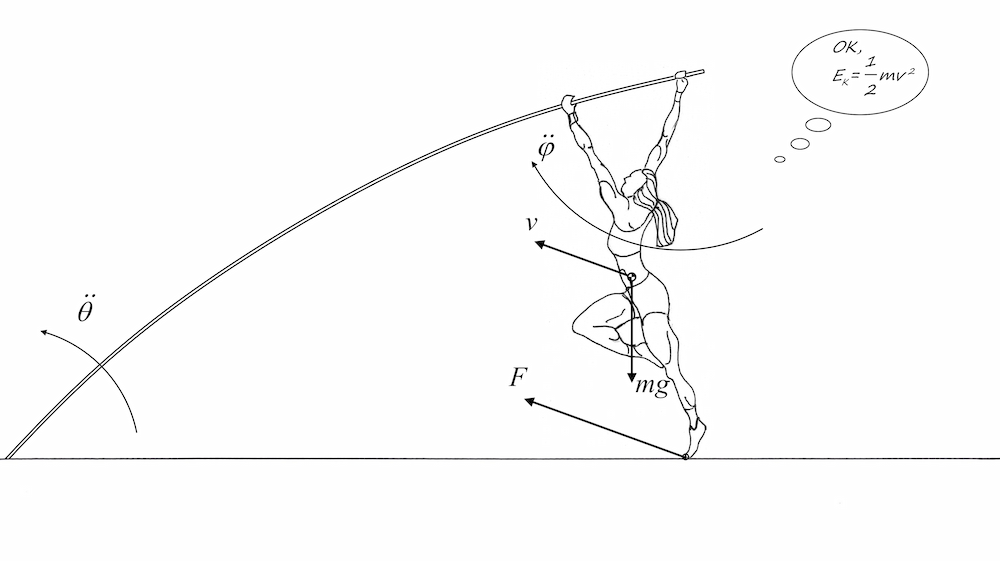
The take-off phase. The double dots denote rotational acceleration.
Let’s now walk through a few pole vaulting scenarios.
At a high angle of attack — when the pole vaulter’s body is straight, with arms stretched and hands held high in the air — the torque leverage, the distance between the ground and the hand grip, is maximized. As a result, the pole rotates forward. If an athlete bends his or her arms, the leverage might not be sufficient enough to produce the amount of torque needed to drive the pole vaulter forward. Because of this, the pole will not reach the vertical position; instead, it will spring the athlete back to the runway. The same situation will occur if the speed of the athlete is not fast enough.
The grip height has a major influence on the take-off phase as well. On one hand, with increasing grip height, the pole vaulter will come higher up along the pole in its straight vertical position. On the other hand, an increased grip height will result in a lower angle of attack, while also increasing the horizontal distance between the pole plant and the body mass, which is the leverage of the counteracting torque from the body mass. However, as an athlete becomes stronger and faster, it is possible to increase the angular momentum, compensating for the additional counteracting torque due to higher grip height.
To maximize the energy transfer to the pole, it is also important that the athlete has a pretensed body. With a looser trunk, as well as shoulders and arms, some of the energy will be dissipated in the body. Body tension has a strong influence on the variables of the pole rotation as well. At takeoff, the athlete pushes backward with his or her leg and generates a forward-acting force. The pole counteracts, rotating the athlete backward. With a loose body, the pole vaulter will come down further on the runway, closer to the pole, and tilt backward. Such a position not only gives the athlete a smaller angle of attack, but it creates a lower jump force and velocity as well — all of which reduce the desired forward rotation of the pole.
At takeoff, the pole vaulter jumps up. This results in a vertical upward and horizontal forward velocity and force. If the angle of the jump is too low, the forces on the pole will bend it substantially. Once the tensile strength of the material has passed, the pole will snap, sending the athlete straight into the landing mat and unfortunately, below the bar. The most common reason for a pole to break is surface damage. When a pole is thrown on the ground or stepped on by track spikes, surface scratches can develop. These small surface marks can be large enough to initiate a pole fracture. Since the materials used in poles (carbon fibers and fiberglass) are brittle, they have a poor tolerance to damage.
Pole Bend and Swing
Once an athlete has jumped, he or she can no longer utilize the runway that previously helped to increase the kinetic energy and counteract the initial pole bending. In this phase, the athlete rotates around the hand grip on the pole, φ, and generates a centripetal force, FC, which further bends the pole. Since the elastic energy of the pole, ES, depends on the deformation of the pole, δ, a higher elastic potential energy is transferred over to the next phase. Further, with greater bending, a higher spring force is stored in the pole. Note that the amount of stored energy and spring force is limited by the material strength.
As we discussed earlier, too much bending of a pole can cause it to break. An athlete can opt to use a pole with a higher stiffness, k, to increase the energy and force, but a stiffer pole exerts a greater stress on the body during the pole plant and takeoff.
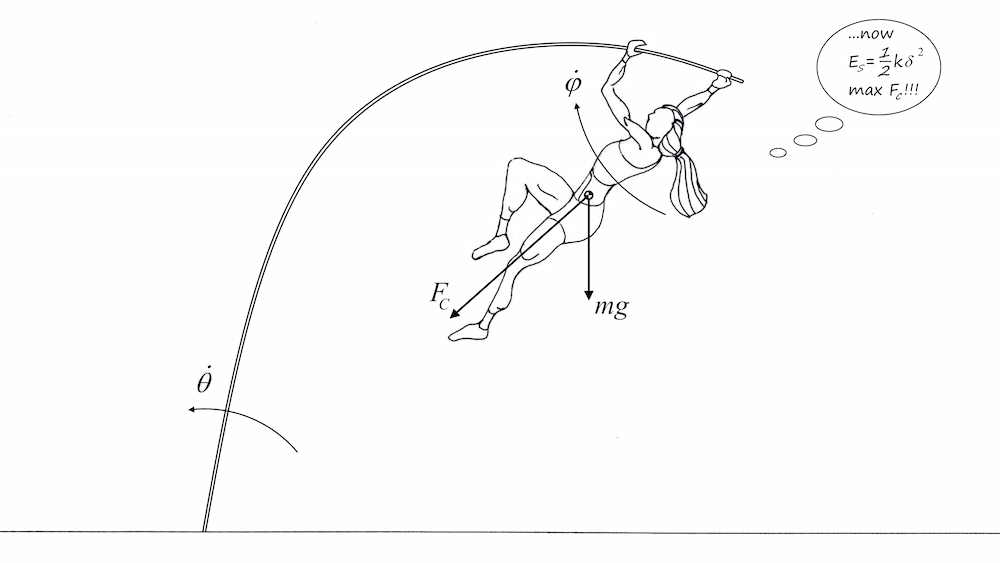
Bending of the pole. The dots indicate rotation velocity.
During the swing, a pole vaulter lifts his or her legs, followed by the torso, to place them above the head when the pole reaches an upright position. The motion reduces the radius between the center of mass and the hand grip, thus increasing the rotation around the hand grip on the pole and sending the athlete higher up into the air. Moreover, the spring force from the pole now comes into play, as it catapults the pole vaulter upward.
With the ability to position the body in a certain shape, an athlete can control the inertia and position of the center of mass. Since both variables affect the angular motion around the hand grip, the athlete can optimize the angular motion of the pole; the elastic energy stored in the pole; and the spring force in the pole (theoretically, the sequence of motion that prompts an increase in jumping height). This involves considering several variables, from the position of multiple body parts to the dynamics of the pole vault. In reality, a pole vaulter’s body must respond to the dynamic changes during the vault, and with perfect timing.
Pull and Release
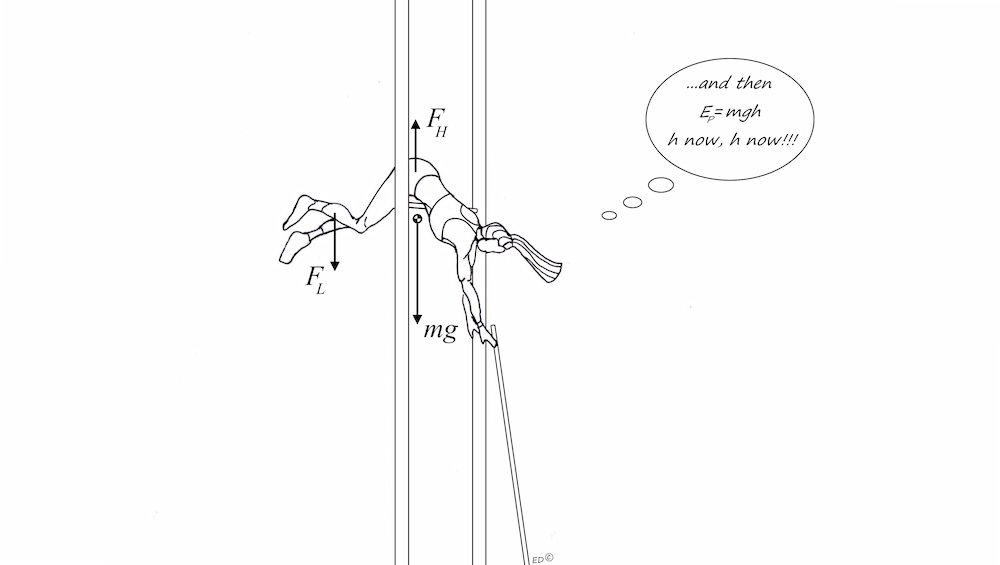
When the pole is in an upright position, muscular energy and the arms are used to pull the body higher up. The velocity of the pull affects the generated power and the work done by the athlete. By increasing the velocity, more work is added to the potential energy at the grip height. This increases the potential energy of the pole vaulter, EP, and therefore enables the clearing of heights above the grip height, h. The timing of such motion is of crucial importance. With an early pull, the athlete will not make it to the bar; with a late pull, the athlete will fly into the bar.
Clearance
From the point at which the athlete releases the pole, he or she is moving as a free body, with the center of gravity following a parabolic path. The initial velocity is mainly directed upward and the gravitational force is acting downward. The pole vaulter’s legs clear the bar. As they are pulled downward, the legs generate a downward force, FL, which is assisted by Newton’s third law of motion. As this happens, the hips are influenced by a counteracting upward force, FH, and the pole vaulter ends up in an upside-down “U” shape. In this configuration, the athlete’s center of mass can pass below the bar, with the body soaring above it. On the way down, Newton’s third law is reused. The athlete moves his or her hips forward, stretching the arms and legs backward so that the upper body can clear the bar.
A Brief Energy Analysis
In a simple analysis of the pole vault, all of the kinetic energy from the run is transferred to the potential energy at clearance. The kinetic energy is E_K= \frac{mv^2}{2}. Here, m is the mass of the athlete and v is the velocity. The potential energy, meanwhile, is E_P= mgh, where g is the acceleration of gravity and h is the height of the elevation. A perfect energy conversion results in a maximum achievable height difference for the center of mass: \Delta h = \frac{v^2}{2g}.
An elite male athlete can reach 9.5 m/s during the run up, while an elite female athlete can reach 8.4 m/s. This corresponds to \Delta h = 4.5\, \mathrm m and \Delta h = 3.5 \, \mathrm m, respectively. Since the center of mass is initially about 1 m above the ground, it is evident that even a perfect conversion of kinetic energy into potential energy brings the pole vaulters to 5.5 m and 4.5 m, respectively. In reality, the best male athletes clear about 6 m and the best female athletes clear about 5 m. The athlete’s muscles supply additional energy during the jump.
Pole Vaulting: A Balance Between Physics and Strength
Pole vaulting consists of many phases. By improving the details behind the technique, centimeter by centimeter and inch by inch, an athlete can work their way up to the limitations of the laws of physics and muscular strength. For many elite athletes, however, such success comes after more than 15 years of training.
Typically, there are two approaches to developing a successful jumping technique. Some people believe that a certain jumping sequence is the perfect approach and thus try to mimic it. Others, however, do not believe that one jumping sequence is the best option for everyone. Instead, they set out to develop their own technique. Incremental improvement can help athletes find local maximum in their height clearance, but to reach higher levels, they must make a significant change. Coping with this modification, which introduces a different response on an athlete’s body, requires the pole vaulter to not only be mentally and physically strong, but also to have a feeling for the physics underlying the sport.
Resources for Learning About the Physics Behind Sports
- Interested in modeling pole vaulting? Read more about the Multibody Dynamics Module and the Structural Mechanics Module, which can be coupled to model such mechanisms in COMSOL Multiphysics.
- You can find several other blog posts pertaining to the physics of sports on the COMSOL Blog. Have a look here.



Comments (0)