Blog Posts Tagged Semiconductor Module
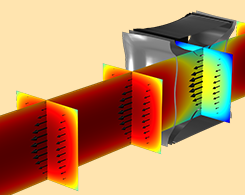
Three Semiconductor Device Models Using the Density-Gradient Theory
You can use the density-gradient theory to model semiconductor devices. Here are 3 examples: a Si inversion layer, Si nanowire MOSFET, and InSb p-channel FET.

Intro to Density-Gradient Theory for Semiconductor Device Simulation
The density-gradient theory is a computationally efficient way to include quantum confinement in the conventional drift-diffusion formulation commonly used for simulating semiconductor devices.
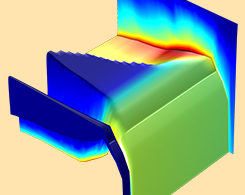
Simulating Radiation Effects in Semiconductor Devices
Analyzing radiation effects in semiconductor devices is an important capability for consumer electronics, medical imaging, nuclear engineering, aerospace, and a wide range of other industries.
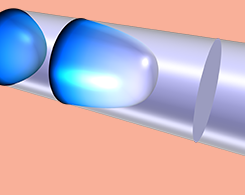
Hydrodynamic Thermal Transport in the Kinetic-Collective Model
F. Xavier Alvarez from the Universitat Autònoma de Barcelona (UAB) discusses using COMSOL Multiphysics® to model heat transfer at the nanoscale and better understand transport processes.

How to Simulate the Carrier Dynamics in Semiconductor Devices
Learn how to simulate carrier dynamics in semiconductor devices with 2 examples: reverse recovery and forward recovery PIN rectifier models.

How to Model the Interface Trapping Effects of a MOSCAP
Looking to analyze interface trapping effects in a MOSCAP? Learn how to use a feature in the Semiconductor Module that enables you to add charging and carrier capture/release effects to a model.

Simulating the Tunneling Current Across a Graded Heterojunction
Interested in semiconductor design? Get an intro to the theory behind quantum tunneling as well as a demonstration of simulating the tunneling current across a graded heterojunction.

Self-Consistent Schrödinger-Poisson Results for a Nanowire Benchmark
This benchmark model of a GaAs nanowire validates the Schrödinger-Poisson Equation multiphysics interface, which is useful for modeling systems with quantum-confined charge carriers.
