Presentazioni e Articoli Tecnici

INTRODUCTION: Point-Of-Care (POC) and home care systems are of growing importance in biotechnological and medical applications [1]. These devices allow health monitoring directly at home or at the doctor’s office. Portability, low-cost, easy handling and compatibility with telemedicine are some of their main advantages. These requirements favor capillary solutions which are usually compact, easily fabricated and highly portable.

In such systems the knowledge of the flow dynamics is very important to handle properly the samples. The dynamic of capillary flow have been studied since the 1920s for cylindrical channels [2]. Studies dealing with non-cylindrical uniform geometries [3] have recently been done still for Newtonian flow. However biological fluids —such as blood—often show non-Newtonian characteristics and modern devices have complex geometries. It is investigated here how COMSOL Multiphysics® software can contribute to the understanding and development of capillary flows in biotechnology and medicine.

USE OF COMSOL MULTIPHYSICS: COMSOL Multiphysics is used with the laminar 2-phase flow level set method available in the Microfluidics Module. The wetted wall condition is used to provide the capillary motion while the inlet and outlet condition are set to a pressure of 0 (corresponding to spontaneous capillary flow conditions). Two approaches are used to analyze the results: Either we integrate the level set variable along a line, which gives the position of the interface, either we average the velocity field along the initial interface, which gives the average velocity of the flow.

RESULTS: Simulations with COMSOL have been used to simulate the dynamic of the spontaneous capillary filling of different microchannels.

First, a square root dependency of the penetration distance according to time is found (Figure 1), in agreement with the Lucas-Washburn-Rideal law. However the velocities obtained with the COMSOL simulations are ~1.5 to 2 times higher than the ones predicted by the theory. To optimize these results, the implementation of the dynamic contact angle is currently under investigation.

Second, we have used COMSOL to simulate a non-uniform geometry consisting of narrow and large segments alternately (Figure 2a). As predicted by the theory, the velocity increases when the advancing interface passes through a narrow segment, and decreases within the larger segments (Figure 2b).

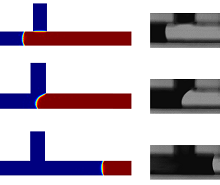
On the other hand, geometry effects such as stop valves, resulting from a pinning phenomenon, have also been simulated with COMSOL (Figure 3).

Finally, 3D simulations of capillary flow with COMSOL show the formation of capillary Concus-Finn filaments [4] in situations where such filaments are predicted by the theory, i.e. in sharp corners for small contact angles.

CONCLUSION: As a conclusion we have shown that the Microfluidics Module of COMSOL Multiphysics can contribute to the understanding of capillary flows in a qualitative manner for uniform and non-uniform channels, and to the prediction of the effect of geometry on capillary flows. In addition, by working on the dynamic contact angle, efforts are made to obtain better quantitative results for the velocities and avoid an overshoot.