Hydrodynamic Heat Transport Model for Semiconductors with Complex Geometries Including Interfaces
The development of new experimental techniques designed to measure the thermal response of semiconductors at reduced length and time scales is revealing the requirement of generalized heat transport models beyond the classical Fourier law [1,2].
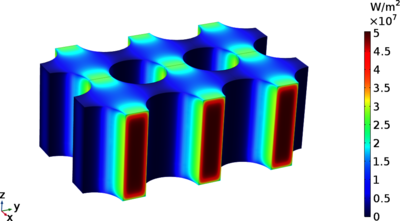
We present the Kinetic Collective Model (KCM), consisting in the hydrodynamic heat transport equation with ab initio calculated coefficients and the corresponding boundary conditions [3,4]. The model equations have been implemented in the weak form using COMSOL Multiphysics® to predict the thermal response of electronic devices with complex geometries including interfaces at reduced length and time scales.
We
compare the model predictions with experimental data on Frequency
Domain Thermoreflectance Experiments (FDTR) in Silicon and with the
effective thermal conductivity of Silicon thin films and holey films
[3]. New phenomenology as phonon viscosity and vorticity arise from
the transport equation by analogy with the Navier-Stokes equation for
fluids, wich allow new physical insight of the non-Fourier effects
taking place at the nanoscale due to boundaries and interfaces with
arbitrary geometry.
[1]
A. Ziabari et.al., Nat. Comm.
9, 255 (2018).
[2] K. M. Hoogeboom-Pot et. al., PNAS 112 16 4851 (2015)
[3] A. Beardo et al. Phys Rev. Applied 11, 034003 (2019)
[4] P. Torres et al. Phys Rev. Materials 2, 076001 (2018)
[4] Y. Guo et. al., Phys. Rev. B 93, 035421 (2018)
[5] R.A. Guyer et. al. Phys. Rev. 2, 148 (1966)