Presentazioni e Articoli Tecnici

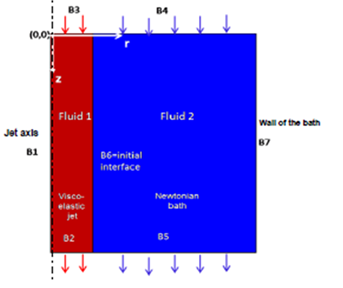
The extensional flow of viscoelastic flow is encountered during fibre spinning processes. A variety of polymers such as polyesters, polyacrylonitrile, rayon, acrylic, spandex etc are manufactured using this process. In the spinning process, polymer melt/ solution is extruded in the form of jet, through a porous plate called spinneret, into a bath having a suitable environment which allows solidification of the polymer to form a fibre. This fibre is drawn by a winder at the exit of the bath, which exerts an extensional stress on the jet, thereby orienting the polymer chains along the fibre axis. This is essential for improving mechanical strength of the fibre. Hydrodynamics of polymeric jets involves extensional flow of viscoelastic fluids. Further complexities are introduced due to change in the rheological properties of the fluid in the jet due to heat and mass transfer. Understanding of hydrodynamics is important for optimizing the process parameters. COMSOL Multiphysics software is ideal suited to address these complexities. In the present work, we have studied velocity profile, interface profile, stress distribution in a cylindrical jet of a viscoelastic fluid in a bath of a Newtonian fluid. The jet is subjected to an extensional stress of known magnitude at the exit of the bath. The jet-fluid is a modeled as an Oldroyd-B fluid. Isothermal operation is considered. The COMSOL simulation is conducted in a 2D axisymmetric geometry. Equation of continuity and motion are solved. The profile of the jet is evaluated using the phase-field model.Simulations are performed for different values of the extensional stress and the Weisenberg number. USE OF COMSOL Multiphysics: We used laminar two phase flow, phase field and PDE interfaces in COMSOL Multiphysics to simulate the flow and incorporate the viscoelastic behaviour. The geometry and the boundary conditions of the problem are shown in Figure 1. We solve both continuity as well as equation of motion in both jet and the bath domains. The interface is traced using phase field equation. For the bath fluid, we use incompressible Newtonian equations and for the jet fluid, we append the Navier Stokes equations for the solvent phase by an extra force term which is a divergence of the stress tensor arising due to presence of the polymer. The extra stress tensor is based on Oldroyd-B constitutive relation written in the convected form. The extensional stress is introduced on the jet, at the exit boundary and is varied to find its effect on the stress distribution in the jet. RESULTS: The solution obtained from the phase field method at 4 different time steps is shown below (Figure 2). The jet fluid (indicated by the deep red color) is cylindrical in the beginning but undergoes progressive contraction along its axis with time. CONCLUSION: This study will pave the way to deeper analysis of spinning process. More complications such as heat and mass transfer and phase change can be superimposed using multiphysics mode of COMSOL.