Presentazioni e Articoli Tecnici

We present a detailed 2D COMSOL Multiphysics^® model used to determine and improve the conversion efficiency in a liquid-metal microfluidics-based portable energy transducer. To date, most work on power generation technologies has focused on the optimization of existing technologies, and relatively few fundamentally new approaches are being explored. In an effort to address this need, we are currently developing a novel class of miniaturized devices able to generate electrostatic energy from mechanical power sources like human locomotion.

The LIMMPET (LIquid Metal Microfluidic Portable Energy Transducer) device operates as a dynamical electrostatic charge amplifier and is realized in the form of a liquid-metal microfluidic architecture. Parallel microfluidic channels carry an alternating pattern of insulating and conducting droplets. In the presence of one or more charged mercury droplets, capacitive interactions with uncharged mercury droplets in the adjacent channel drives charge separation through external “charge bridge” electrodes, shown in Figure 1b, resulting in exponentially growing charge accumulation on neighboring droplets. This charge is then collected by output terminals, which can be connected to a load for immediate use, or to a capacitor for storage. Theoretical analysis suggests the LIMMPET is capable of producing 12µW at an unparalleled conversion efficiency of over 95%, a tenfold improvement over current metrics.

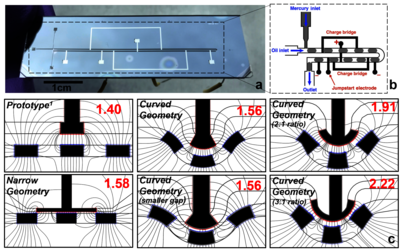
To date, we have demonstrated the feasibility of this novel power generation concept using a bonded PDMS-glass [AE1] device, however, detailed analysis of the of our initial prototype suggests that the linear—instead of exponential—voltage growth is due to insufficient capacitive coupling between droplets.¹ To characterize LIMMPET performance, we define the ratio of the charge induced on a droplet Q_f to the charge of the inducing droplets in the adjacent channel Q_i as the charge amplification factor, Γ=Q_f ⁄ Q_i. This parameter can be calculated analytically for simplified geometries of alternating conducting and insulating droplets by solving a system of linear equations Q=CV where Q is the charge on a droplet, C is the Maxwell capacitance matrix, and V is the droplet potential. For the existing prototype geometry (PDMS-glass), such calculations indicate that Γ≅0.4; exponential growth requires Γ>1.

To inform our design process—with the ultimate goal of maximizing —we model the dynamic process of charge-induction on the droplets in COMSOL Multiphysics^® simulation software. We couple the Electric Currents equations (AC/DC Module) for charge conservation to those governing Laminar Two-Phase Flow (CFD Module), using a phase field formulation to capture the hydrodynamic generation of droplets and thereby define the interface between the conductive and insulating materials; due to nonuniform current densities induced within the two phases by interactions with moving charged droplets, charge accumulates at this diffuse interface (shown in red and blue in Figure 1c). Results from our model have provided invaluable physical insight into approaches for facilitating the capacitive coupling between adjacent droplets. Most importantly, the discovery that narrow, curved geometries significantly improve capacitive interaction, led to the subsequent predicted charge amplification ratio of Γ=2.22. Development is currently underway to fabricate and test this improved design.